What the equation means is that there exist constant $A>0$ and $B,C$ such that $$ |x| \leq A \Longrightarrow Bx^2 \leq e^x-1-x \leq Cx^2. $$ In particular, for small $x$, $e^x$ is very close to $1+x$, since the error is only $O(x^2)$ (when $x$ is small, $x^2$ is much smaller than $x$).
The same estimate doesn't hold for large $x$ — indeed, $e^x$ grows faster than $x^2$, indeed faster than any fixed power of $x$. So the quoted estimate only holds for small $x$.
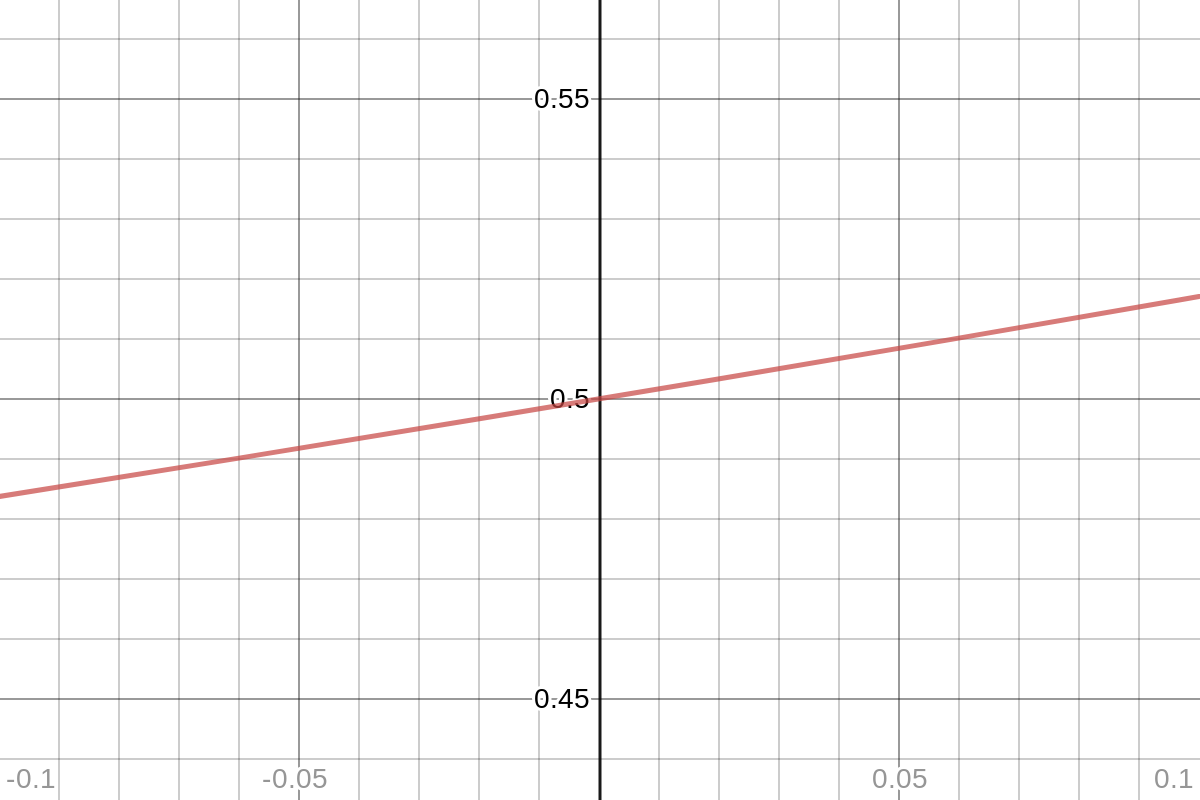
Here is a graphic example. Below is the plot of $\frac{e^x-1-x}{x^2}$ for $|x| \leq 0.1$. You can see that it is very close to $0.5$. This is consistent with the Taylor expansion of $e^x$, which is $1 + x + x^2/2 + O(x^3)$.
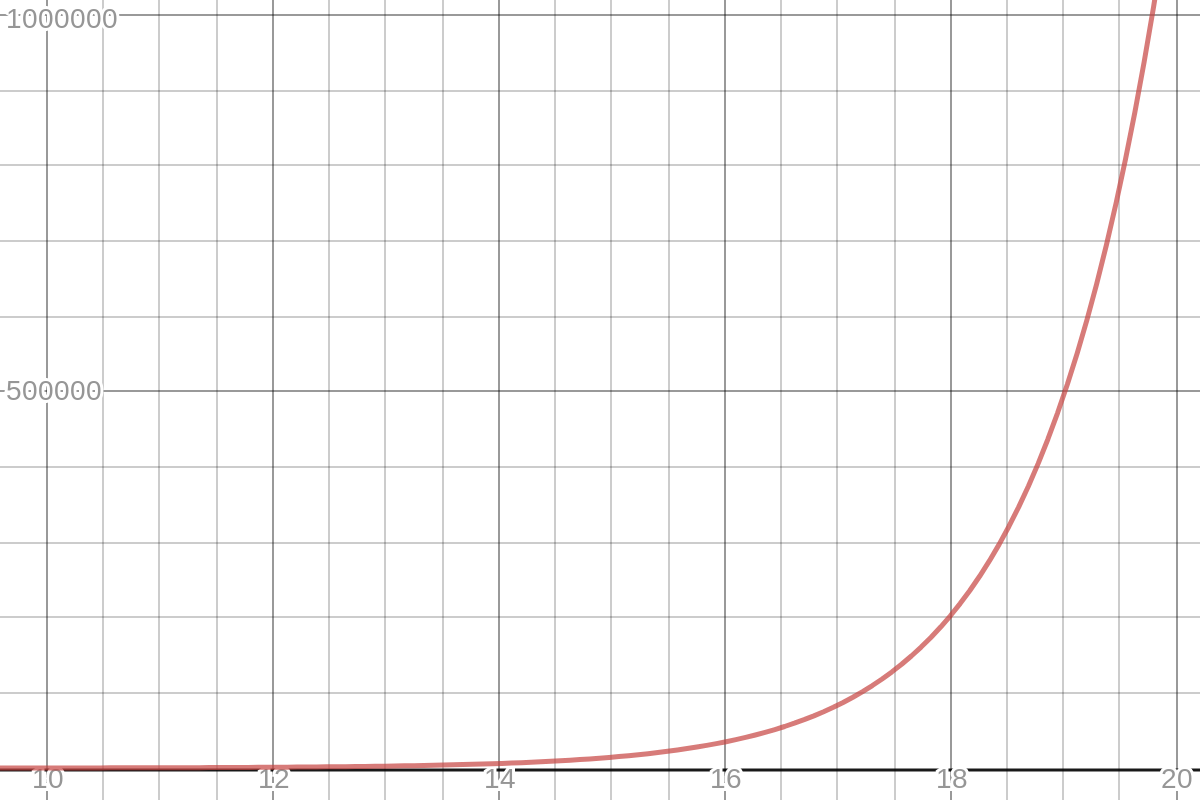
In contrast, here is the same plot for $10 \leq x \leq 20$. You can see that the ratio shoots up to infinity.
Credit: plots executed using Desmos.