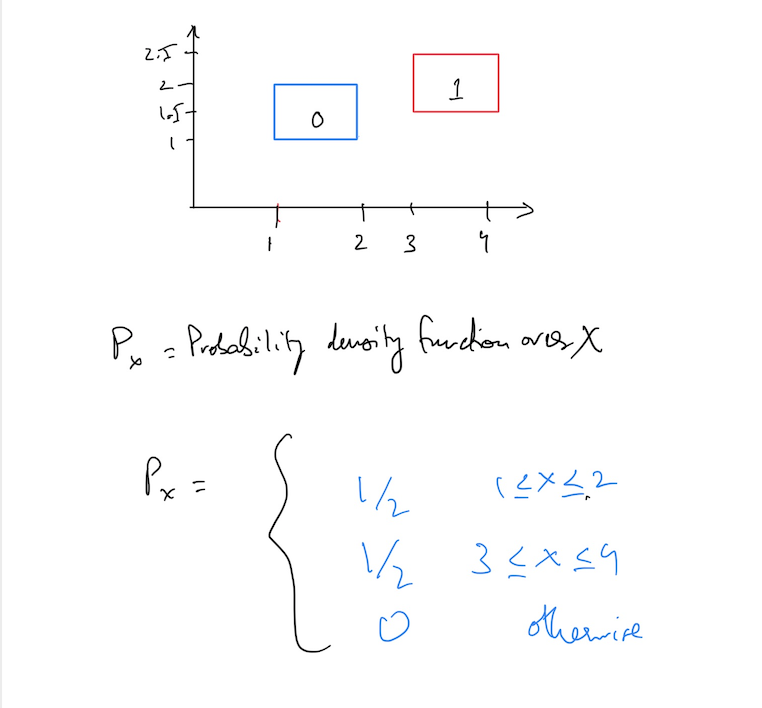
A probability distribution $P$ over $X \times \{0, 1\}$. $P$ can be defined in term of its marginal distribution over $X$ , which we will denote by $P_X$ and the conditional labeling distribution, which is defined by the regression function $$ \mu(x) = P_{ (x,y) \sim P} [y = 1 \mid x] $$ Consider a 2-dimensional Euclidean domain, that is $X = \mathbb R^2$, and the following process of data generation: The marginal distribution over $X$ is uniform over two square areas $[1, 2] \times [1, 2] \cup [3, 4] \times [1.5, 2.5]$. Points in the first square $Q_1 = [1, 2] \times [1, 2]$ are labeled 0 (blue) and points in the second square $Q_2 = [3, 4] \times [1.5, 2.5]$ are labeled 1 (red).
Describe the density function of $P_X$, and the regression function, Bayes predictor and Bayes risk of $P$.
In the image, I have defined the Probability density function. Is that correct? I want to find it over both the axis andam having trouble in generalfiguring out the pdf of this function in two dimensions2 dimensional space.