I'm late for the party, but anyway... Let $p$ and $q$ be two points, such that the distance $d$ between them is maximum over all the pairs of points in the given point set. All the other points must be inside the intersection of two disks with radius $d$ - one disk with center in the point $p$ and another disk with center in the point $q$. This intersection looks like a curvilinear rhombus with diagonal $(p, q)$ and circular sides with radius $d$.
Now
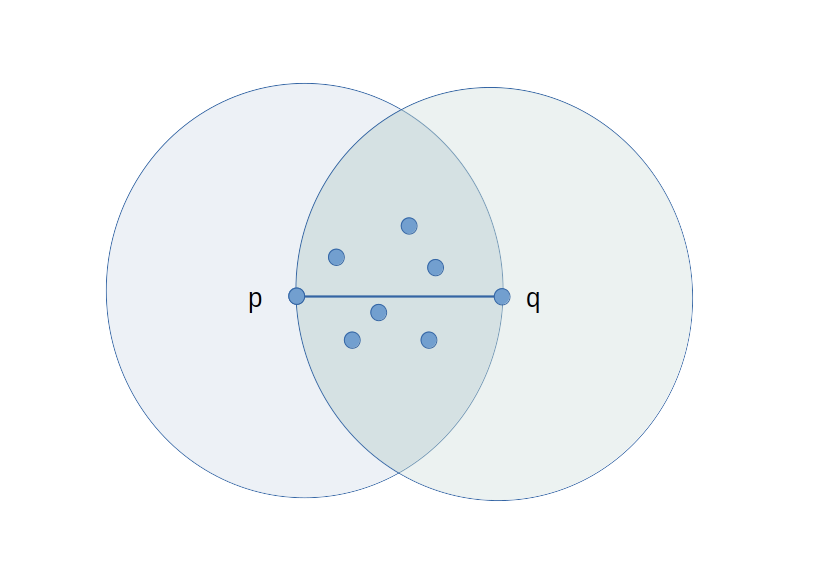 Now we'll use the observation from the @Joe answer - the farthest point in any direction belongs to the convex hull. In our case this direction is defined by the line, passing through points $p$ and $q$, and these two points are obviously farthest along this direction.
Now we'll use the observation from the @Joe answer - the farthest point in any direction belongs to the convex hull. In our case this direction is defined by the line, passing through points $p$ and $q$, and these two points are obviously farthest along this direction.
