Closest-point heuristic approximation problem

Question

I have an Approximation Algorithm problem and its answer but I am having a hard time to clearly understand.
The problem is as follows:

Consider the following closest-point heuristic for building an approximate traveling-salesman tour whose cost function satisfies the triangle inequality. Begin with a trivial cycle consisting of a single arbitrarily chosen vertex. At each step, identify the vertex $u$ that is not on the cycle but whose distance to any vertex on the cycle is minimum. Suppose that the vertex on the cycle that is nearest $u$ is vertex $v$. Extend the cycle to include $u$ by inserting $u$ just after $v$. Repeat until all vertices are on the cycle. Prove that this heuristic returns a tour whose total cost is not more than twice the cost of an optimal tour.

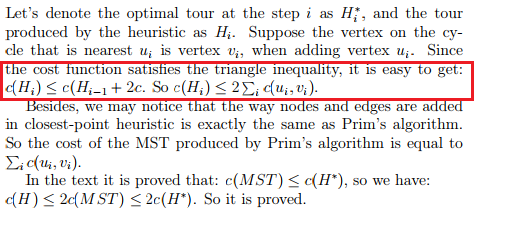
The answer is (I apoligize for including an image but I couldn´t get the some special characters displayed correctly)

What I do not understand is the part surrounded by the red rectangle. I will very much appreciate if you help me to get the idea behind it. I am studying for an exam and I am stuck with this problem.

Respectfully,
Jorge Maldonado

Answer 1

I think there's some problem with the LaTeX formula. It should look like this:

$c(H_i)\leq c(H_{i-1})+2c(u_i, v_i)$

Let's say initially it was $u\to x$. Then you add $v$ directly after $u$, forming $u\to v\to x$. This increases the total distance by $c(u,v)+c(v,x)-c(u,x)$. From the triangle inequality, we have $c(u,v) + c(u,x) \geq c(v,x)$ and thus $2c(u,v)\geq c(u,v)+c(v,x)-c(u,x)$, hence $c(H_i)\leq c(H_{i-1})+2c(u_i, v_i)$.

The next paragraph should make more sense now.