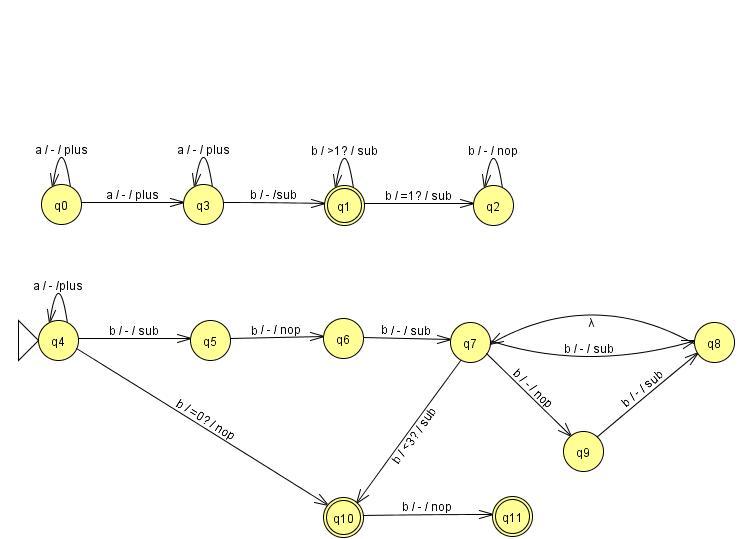
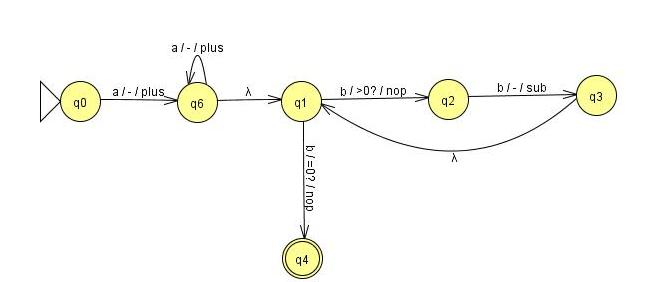
Let $A= \{L \mid L \;\text{is one-counter and \(\bar{L}\) is also one-counter} \}$
Clearly, $\text{Deterministic one-counter} \subseteq A$
Is it the case that $ A = \text{Deterministic one-counter}$?
I know that for context-free languages the analogue is not the case. For example, let $P =\{ ww^r\}$. Then both $P$ and $\bar{P}$ are context-free but $P$ is not deterministic. Hence $A$ defines a (strict) subset of the context-free languages.
The question is: can we construct a similar one-counter example for which the same holds?