I'm really stuck here and do not know what to do. So far, I've constructed a DFA and a regular expression that produces the aforementioned set of strings. Namely, the DFA looks like: 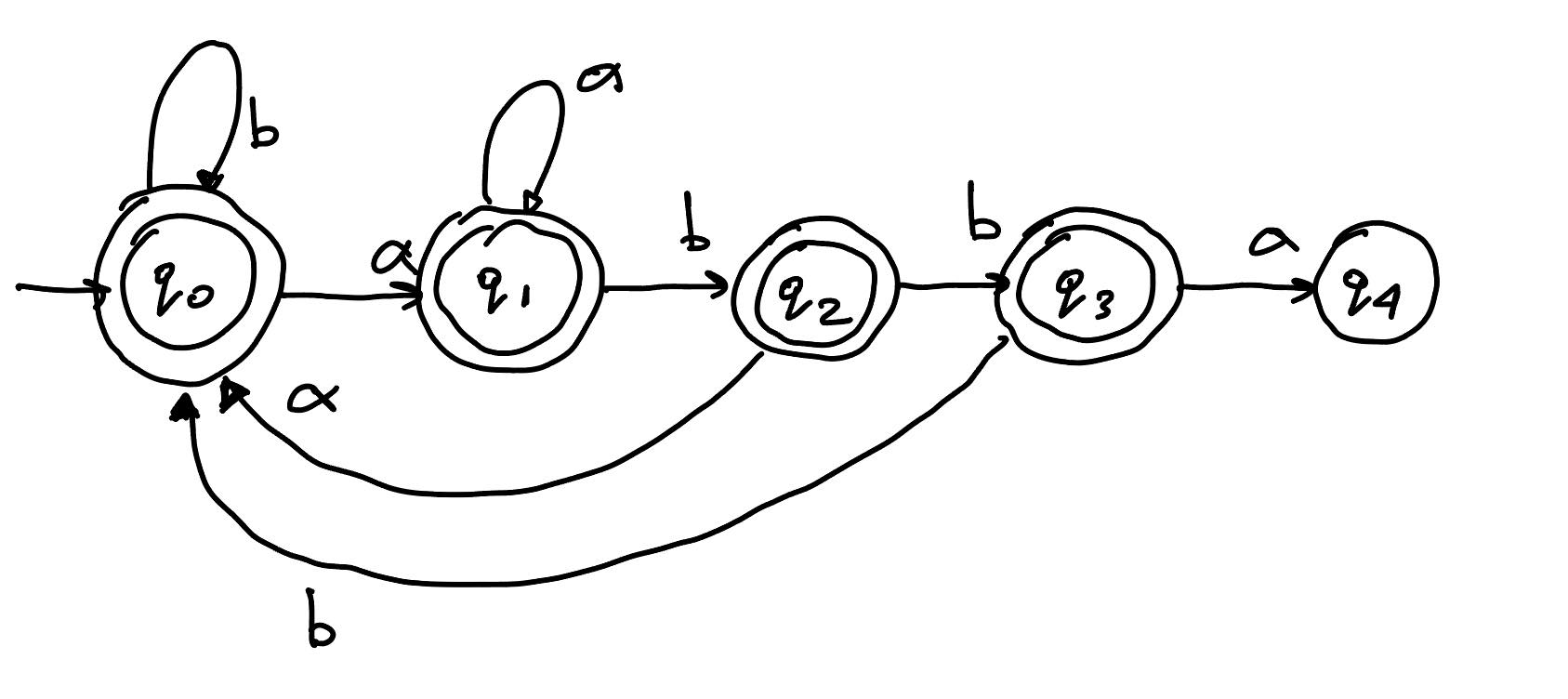
After a lot of efforts I managed to find a concise regular expression of it, namely: $$ b^*\left( a + (b \; | \; bbb+)\right)^*a^*b^*.$$
What I have tried so far as to construct the desired regular grammar is: \begin{align*} q_0 &\rightarrow b \ | \ a \ | \ aq_1 | \ ε \ \\ q_1 &\rightarrow aq_1 \ | \ bq_2 \ | \ ε \ \\ q_2 &\rightarrow aq_0 \ | \ bq_3 \ | \ ε \ \\ q_3 &\rightarrow bq_0 \ | \ aq_4 \ | \ ε \end{align*}
Obviously $q_4$ is non-yielding, therefore we can omit it, and we get
\begin{align*} q_0 &\rightarrow b \ | \ a \ | \ aq_1 | \ ε \ \\ q_1 &\rightarrow aq_1 \ | \ bq_2 \ | \ ε \ \\ q_2 &\rightarrow aq_0 \ | \ bq_3 \ | \ ε \ \\ q_3 &\rightarrow bq_0 \ | \ ε \end{align*}
Correct me if I'm wrong but the above rules as is, define a context free grammar since we have more than one non-Terminal symbol on the right side. How can I turn the above rules into a regular grammar? Any help would be greatly appreciated.
