Give a DFSA that accepts $L_1 = \{x : \#_{01}(x) \mod 3 = 0\}$ using as few states as possible

Question

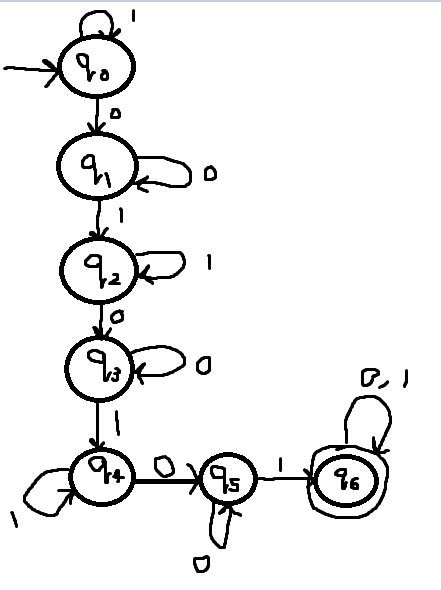
Let $L_1$ be the language over alphabet $\{0, 1\}$ defined by $L_1 = \{x : \#_{01}(x) \mod 3 = 0\}$.

Using as few states as possible, give a DFSA that accepts $L_1$.

Also give an appropriate state invariant for your DFSA.

---

I have a regex for it and that is $1^*(00^*11^*00^*11^*00^*11^*)1^*$.

An example of accepted strings are: 100111010001. Example of a rejected string is 01010101, since |01010101| $= 4 \mod 3 = 1 \neq 0$.

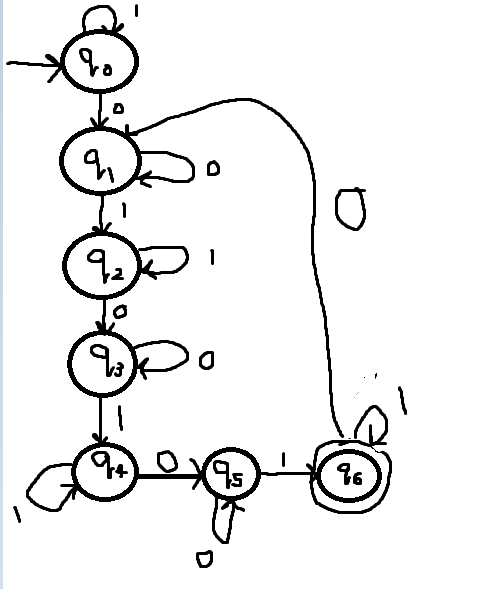
How would I draw it? Also do I come up with state invariant first or draw the dfsa first? I drew the dfsa first right now

100111010001 - gets accepted. However 01010101 also gets accepted when it should be rejected. Not sure how to limit it to just mod 3

Edit:

I believe this works. (I cant use regexes in state invariants)

$q_0$: $x$ must contain only $1$'s

$q_1$: $x$ contains 1's before any 0's and ends with a 0

..

not sure how to write the state invariant for this case

Answer 1

Hint, a correct regular expression is $1^*(00^*11^*00^*11^*00^*11^*)^*0^*$.

There are 6 states, where $q_{0,1}$ is the initial state. Both $q_{0,1}$ and $q_{0,0}$ are accepting states.

$q_{0,1}$: the number of 01s is a multiple of 3 and it ends with 1. Or empty word.
$q_{0,0}$: the number of 01s is a multiple of 3 and it ends with 0.
$q_{1,1}$: the number of 01s is 1 plus a multiple of 3 and it ends with 1.
$q_{1,0}$: the number of 01s is 1 plus a multiple of 3 and it ends with 0.
$q_{2,1}$: the number of 01s is 2 plus a multiple of 3 and it ends with 1.
$q_{2,0}$: the number of 01s is 2 plus a multiple of 3 and it ends with 0.