Suppose we have $n$ lines in plane which is divided equally among the three sets(the lines in each set are equally spaced), each of contains $n/3$ lines. And they intersect each other and creates a triple intersection.
My question is how can I prove that there are $\Theta(n^2)$ triple intersections?
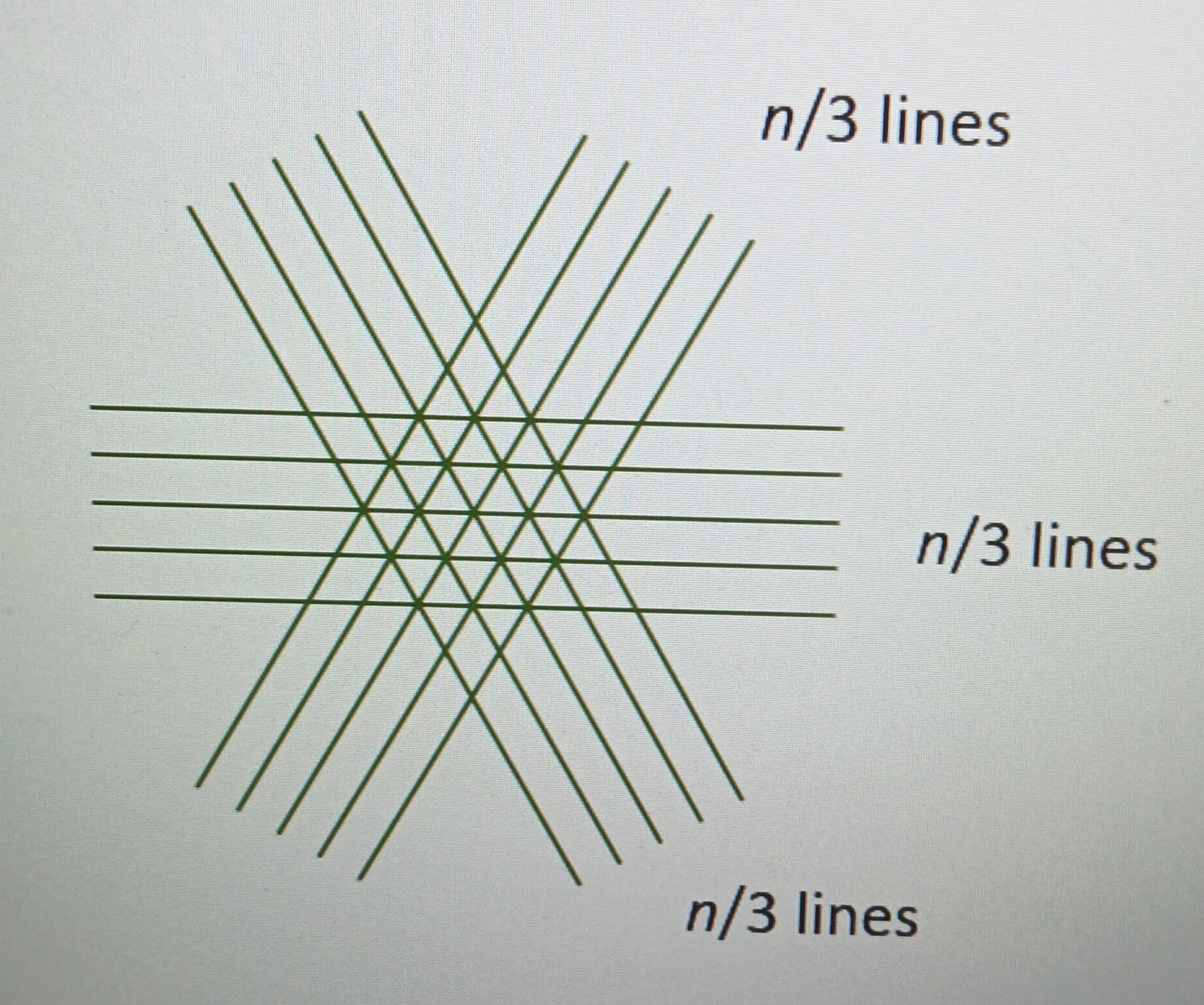
This is in no way formal, it is based on the assumptions and intuition given by the asker in the comments, and under the assumption that $\frac{n}{3}$ is odd. The middle horizontal line $l_0$ crosses $\frac{n}{3} -1$ rhombus, where each pair of parallel edges of a rhombus are induced by two adjacent lines from the same vertical set. The horizontal lines $l_1$ and $l_2$, above and below $l_0$, respectively, cross $\frac{n}{3} - 2$ rhombus each. Proceeding similarly, we get the horizontal line $l_3$ above $l_1$, and the horizontal line $l_4$ below $l_2$ cross $\frac{n}{3} - 3$ rhombus each. Proceeding iteratively, and assuming $\frac{n}{3}$ is odd, we get that the number of rhombus crossed horizontally is $$ (\frac{n}{3} -1) + 2\cdot \sum\limits_{i=2}^{\frac{\frac{n}{3} - 1}{2}} (\frac{n}{3} - i)$$
As the the number of triples each horizontal lines passed through equals the number of rhombus it crosses plus one, we get that the number of triples is
$$ \frac{n}{3} + 2\cdot \sum\limits_{i=2}^{\frac{\frac{n}{3} - 1}{2}} (\frac{n}{3} - i + 1) = \theta(n^2)$$
The lines define a tiling of a regular hexagon with equilateral triangles. If you add two lines symmetrically to all three pencils, the size of the hexagon grows by one layer.
When the number of lines grows linearly, the number of triangles, as well as the number of vertices (by the Euler relation), grows as an area, i.e. quadratically.
