the question is as follows, further the notation used are standard as used in theory of computation $$L = \big\{ w : w \in \{a,b\}^*,\ |n(a) - n(b)| = 2k\}\,.$$
Beware 'K' is not a fixed integer its a set of natural number , so 2k means all natural nunbers divisible by 2 and so on for 3k , 4k... .I think the above language is irregular or its finite automata can't be formed. Firstly the above question in basic language can be thought of as modulus of difference of number of $a$ and number of $b$ is divisible by $2$ or is even , similar to this reasoning we can extend this to more general like $3k$, $4k$, $5k$,... and so on. Secondly we know that any condition which have comprise the difference between the symbols would have infinite possible comparison between symbols, like an equation of $n(a) - n(b) =0/1/2/3...$ needs infinite states of finite automata which makes them irregular.
But here with the use of modulus it seems no different as here also we will need many comparison between symbols.
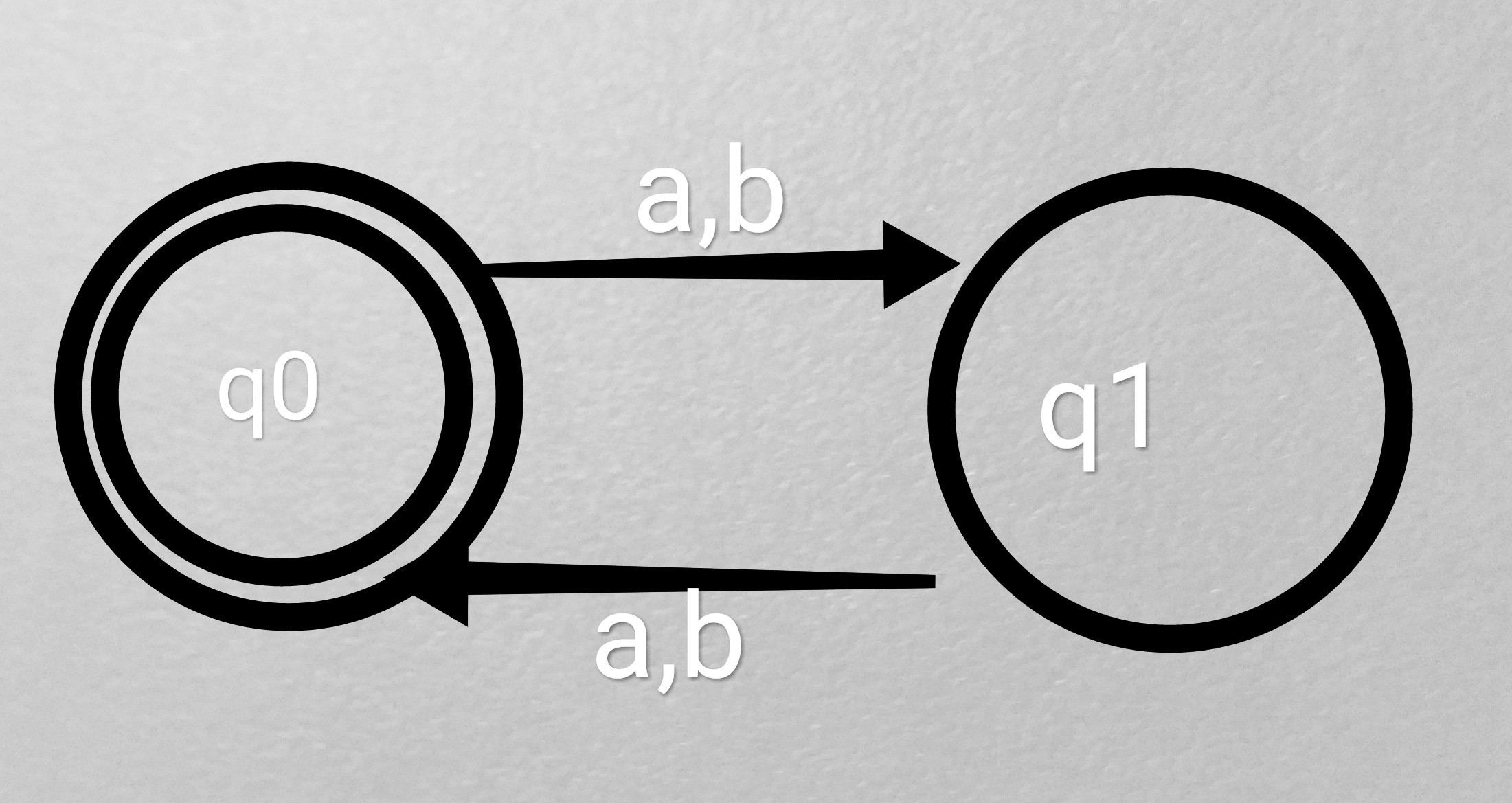
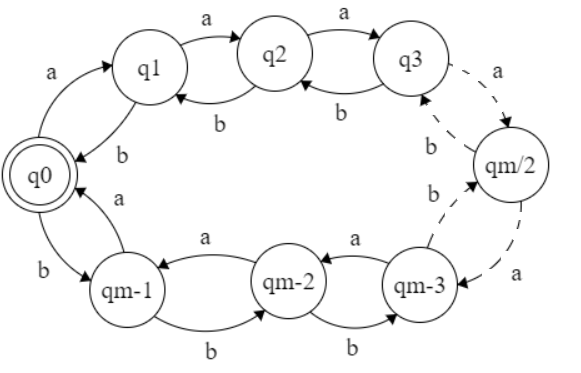
Thirdly its more of a general question like when define regularity of language $L$ over condition like, $n(a) - n(b)\bmod 3 =0$, we say the all strings can be classified as having either remainder $0$,$1$,$2$ which by using Myhill-Nerode theorem proves the language regular. But why cant we use the same analogy over the relation like $n(a) -n(b)=2$ saying the all the strings would either have a difference $2$ or not. Further if you could answer the regularity of $n(a)- n(b) = 2k$, also how does taking modulus effect its regularity and how does it makes the states of finite automata finite which before applying modulus were infinite if you could a graphical representation would be appreciated.