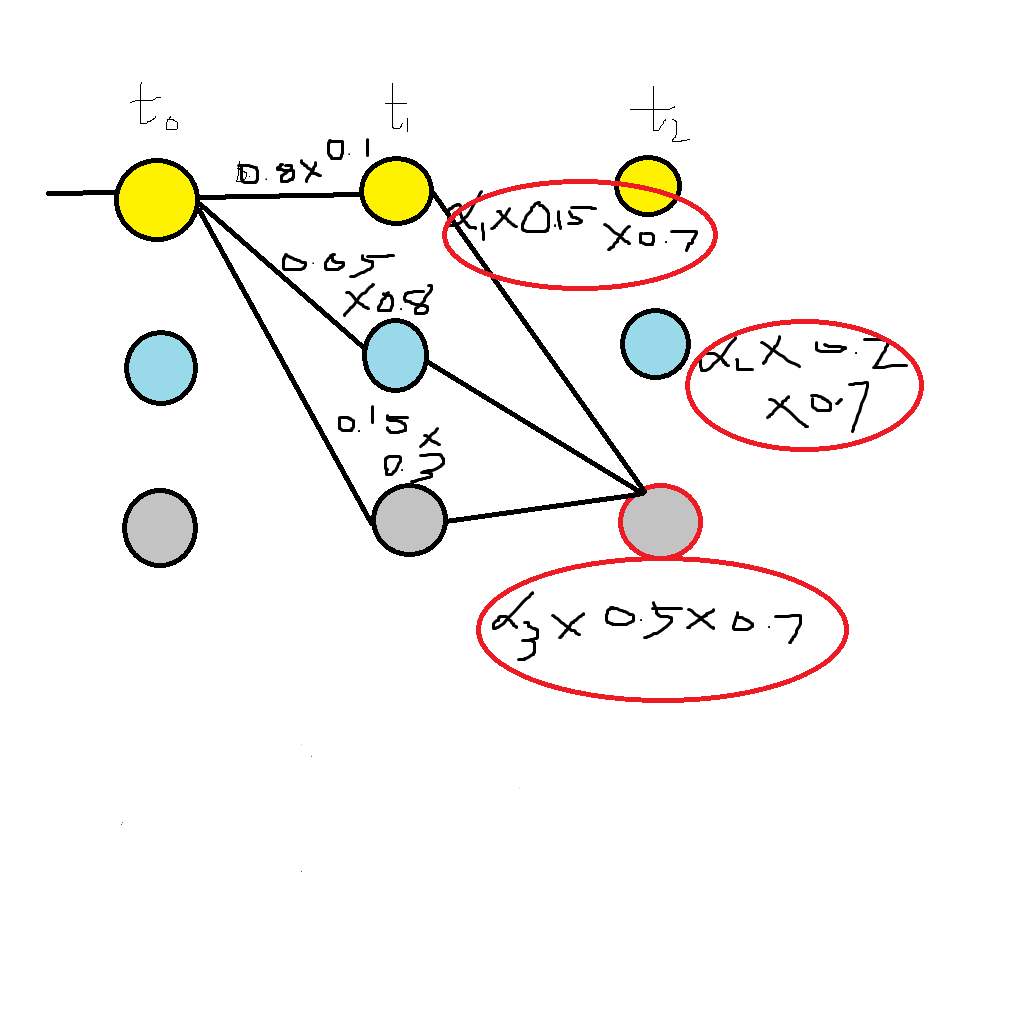
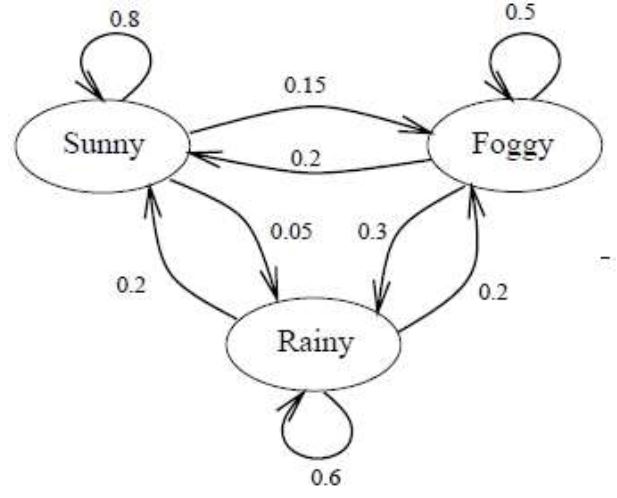
This is the HMM model considered in the question
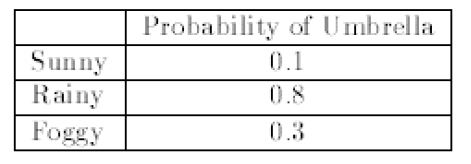
And this is the emission probabilities for the respective states. There are two emission values, bringing an umbrella and not bringing an umbrella.
Description of the question:
The question considered the initial state as sunny. On day 2, the person brought an umbrella. On day 3, the person didn't carry an umbrella.
We have to find the probability that it's foggy on 3rd day.
Given: Prior probability of the caretaker carrying an umbrella is 0.5.
According to me, the answer to this question was addition of the terms in the red circles.
Where α1 = 0.8 * 0.1, α2 = 0.05 * 0.8, α3 = 0.15 * 0.3
Problem I faced:
But the answer included dividing the answer I got by (0.5)2. That is, I guess they are dividing by the prior probability two times for the two transitions, but I'm not clear as to why they are dividing.