Take the image and spectrum below. If I look at the spectrum, it just look like noise.... How to make sense of it intuitively?
Image:
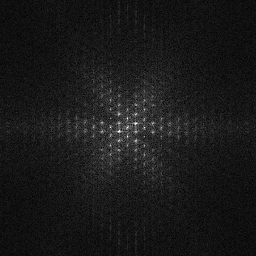
Frequency spectrum of image (using Fourier Transform):
$\begingroup$
$\endgroup$
1
-
3$\begingroup$ Fourier transform is not local. A single point of the transform gives you almost no information about the original function. $\endgroup$– tomaszCommented Jun 9, 2013 at 10:36
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
3
As stated by @tomasz, the fourier transform relates only globally to the image since the pixels of the transform correspond to frequencies in the original image (which you may intuitively understand - somewhat - as speed of color variation as you move on the original image). This is well explained on the web : An Intuitive Explanation of Fourier Theory
Look in particular at the bottom of the page.
-
$\begingroup$ @user8005 - The dots that you see on your transform are caused by the regularity and repetitiveness of the honeycomb on the original image. They say that some spatial frequencies (multiple of honeycomb cell size) are structuring this image. $\endgroup$– babouCommented Jun 9, 2013 at 17:39
-
$\begingroup$ 'caused by the regularity and repetitiveness' how does that make sense intuitively? $\endgroup$– user8005Commented Jun 9, 2013 at 20:46
-
$\begingroup$ The pixels in the transform represent spatial frequencies in the original image. Since the honeycomb structure emphasizes fairly precisely some spatial frequencies, the corresponding pixels get more intense. Read the reference on the web. $\endgroup$– babouCommented Jun 10, 2013 at 12:42