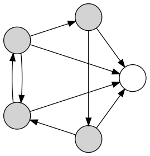
We can eliminate all but one of the vertices by checking for the existence of $n-1$ edges because we can eliminate one possibility for each edge we check. In particular, if there is an edge going from $x$ to $y$, we eliminate $x$ and move on to $y$ (as another vertex can be reached from it); if not, we eliminate $y$ (as it cannot be reached from $x$). Once we reach the last vertex, whichever vertex is not eliminated should be compared with each other vertex (ensure the superstar condition is upheld: there is an edge incoming but not outgoing) until it is eliminated or confirmed as the superstar. Some pseudocode:
vertex superstar(graph g)
current vertex = first
# Go through each vertex
for each subsequent vertex in g ("next")
# If there's an edge from this to the next, we eliminate this one [move to the new one].
# If not, we just stay here.
if edge exists from current to next
candidate = next
end if
end for
# Now we are on the final remaining candidate, check whether it satisfies the requirements.
# just a rename for clarity
candidate = current
for each other vertex in g
if edge from current to other exists
return null
else if no edge from other to current
return null
end if
end for
return candidate
end superstar
Let's walk through an example to illustrate the method. Take this array, with the source vertex on the top and destination on the side. 1 indicates an edge:
$$
\begin{array}{r|c c c c|r}
& 1 & 2 & 3 & 4 \\
\hline
1 & - & 1 & 0 & 1 \\
2 & 1 & - & 0 & 1 \\
3 & 1 & 1 & - & 1 \\
4 & 1 & 1 & 0 & - \\
\end{array}
$$
I'll grey out the vertices we have ruled out as potential superstars.
I'll use green and red to indicate the edges we are looking at when they do and do not contain the edge we're looking for, and blue to indicate where we have already looked.
We start by looking at vertices 1 and 2.
$$
\begin{array}{r|c c c c}
& 1 & 2 & 3 & 4 \\
\hline
1 & - & \color{green}{1}
& 0 & 1 \\
2 & 1 & - & 0 & 1 \\
3 & 1 & 1 & - & 1 \\
4 & 1 & 1 & 0 & - \\
\end{array}
$$
The green number shows there is an
edge from 2 to 1, so we eliminate 2 and look for an edge from 3 to 1:
$$
\begin{array}{r|c c c c}
& 1 & \color{grey}{2} & 3 & 4 \\
\hline
1 & - & \color{blue}{1}
& \color{red}{0}
& 1 \\
\color{grey}{2} & 1 & - & 0 & 1 \\
3 & 1 & 1 & - & 1 \\
4 & 1 & 1 & 0 & - \\
\end{array}
$$
We see there is no such edge, so we eliminate 1 and take 3 as our current
vertex. Recall that we have eliminated 2 already, so see whether there is an edge from 4 to 3:
$$
\begin{array}{r|c c c c}
& \color{grey}{1} & \color{grey}{2} & 3 & 4 \\
\hline
\color{grey}{1} & - & \color{blue}{1}
& \color{blue}{0}
& 1 \\
\color{grey}{2} & 1 & - & 0 & 1 \\
3 & 1 & 1 & - & \color{green}{1} \\
4 & 1 & 1 & 0 & - \\
\end{array}
$$
There is an edge from 4 to 3, so we eliminate 4. At this point we have
eliminated all but one of the vertices (3), so check out its edges and see whether it qualifies:
$$
\begin{array}{r|c c c c}
& \color{grey}{1} & \color{grey}{2} & 3 & \color{grey}{4} \\
\hline
\color{grey}{1} & - & \color{blue}{1}
& \color{red}{0}
& 1 \\
\color{grey}{2} & 1 & - & 0 & 1 \\
3 & \color{green}{1} & 1 & - & \color{blue}{1} \\
\color{grey}{4} & 1 & 1 & 0 & - \\
\end{array}
$$
There is an edge from 1 to 3 but not the reverse, so 3 is still a candidate.
$$
\begin{array}{r|c c c c}
& \color{grey}{1} & \color{grey}{2} & 3 & \color{grey}{4} \\
\hline
\color{grey}{1} & - & \color{blue}{1}
& \color{blue}{0}
& 1 \\
\color{grey}{2} & 1 & - & \color{red}{0}
& 1 \\
3 & \color{blue}{1}
& \color{green}{1}
& - & \color{blue}{1} \\
\color{grey}{4} & 1 & 1 & 0 & - \\
\end{array}
$$
There is also an edge from 2 to 3 but not the reverse, so 3 is still a candidate.
$$
\begin{array}{r|c c c c}
& \color{grey}{1} & \color{grey}{2} & 3 & \color{grey}{4} \\
\hline
\color{grey}{1} & - & \color{blue}{1}
& \color{blue}{0}
& 1 \\
\color{grey}{2} & 1 & - & \color{blue}{0}
& 1 \\
3 & \color{blue}{1}
& \color{blue}{1}
& - & \color{green}{1} \\
\color{grey}{4} & 1 & 1 & \color{red}{0}
& - \\
\end{array}
$$
There is an edge from 4 to 3 but not 3 to 4; that completes our check of 3's edges and we have found that it is, in fact, a superstar.
Since we eliminate one vertex as a possible superstar on each of the first
$n-1$ edge checks, the best case is that the $n$th check eliminates the
final vertex for a complexity of $n$. In the worst case (the last or
second-to-last vertex is a superstar, or the final check disqualifies it), after the first $n-1$ comparisons we compare the candidate
with $2\times(n-1)$ more vertices, for a worst case complexity of $3n-3$ ($O(n)$). So, this algorithm is
$\Theta(n)$.