How to show two models of computation are equivalent?

Question

I'm seeking explanation on how one could prove that two models of computation are equivalent. I have been reading books on the subject except that equivalence proofs are omitted. I have a basic idea about what it means for two models of computation to be equivalent (the automata view: if they accept the same languages). Are there other ways of thinking about equivalence? If you could help me understand how to prove that the Turing-machine model is equivalent to lambda calculus, that would be sufficient.

Answer 1

You show that either model can simulate the other, that is given a machine in model A, show that there is a machine in model B that computes the same function. Note that this simulation does not have to be computable (but usually is).

Consider, for example, pushdown automata with two stacks (2-PDA). In another question, the simulations in both directions are outlined. If you did this formally, you would take a general Turing machine (a tuple) and explicitly construct what the corresponding 2-PDA would be, and vice versa.

---

Formally, such a simulation may look like this. Let

$\qquad \displaystyle M = (Q,\Sigma_I,\Sigma_O,\delta,q_0,Q_F)$

be a Turing machine (with one tape). Then,

$\qquad \displaystyle A_M = (Q \cup \{q^*_1,q^*_2\},\Sigma_I,\Sigma_O', \delta', q^*_1, Q_F)$

with $\Sigma_O' = \Sigma_O \overset{.}{\cup} \{\$\}$ and $\delta'$ given by

$\quad \displaystyle (q^*_1,a,h_l,h_r) \to_{\delta'} (q^*_1,ah_l,h_r)$ for all $a \in \Sigma_I$ and $h_r,h_l \in \Sigma_O$,
$\quad \displaystyle (q^*_1,\varepsilon,h_l,h_r) \to_{\delta'} (q^*_2,h_l,h_r)$ for all $h_r,h_l \in \Sigma_O$,
$\quad \displaystyle (q^*_2,\varepsilon,h_l,h_r) \to_{\delta'} (q^*_2,\varepsilon,h_lh_r)$ for all $h_r,h_l \in \Sigma_O$ with $h_l \neq \$$,
$\quad \displaystyle (q^*_2,\varepsilon,\$,h_r) \to_{\delta'} (q_0,\$,h_r)$ for all $h_r \in \Sigma_O$,
$\quad \displaystyle (q,\varepsilon,h_l,h_r) \to_{\delta'} (q',\varepsilon,h_la) \iff (q,h_r) \to_\delta (q',a,L)$ for all $q \in Q$ and $h_l \in \Sigma_O$,
$\quad \displaystyle (q,\varepsilon,\$,h_r) \to_{\delta'} (q',\$,\square a) \iff (q,h_r) \to_\delta (q',a,L)$ for all $q \in Q$,
$\quad \displaystyle (q,\varepsilon,h_l,h_r) \to_{\delta'} (q',ah_l,\varepsilon) \iff (q,h_r) \to_\delta (q',a,R)$ for all $q \in Q, h_l \in \Sigma_O'$,
$\quad \displaystyle (q,\varepsilon,h_l,\$) \to_{\delta'} (q,h_l,\square\$)$ for all $q \in Q$ and $h_l \in \Sigma_O'$, and
$\quad \displaystyle (q,\varepsilon,h_l,h_r) \to_{\delta'} (q',h_l,a) \iff (q,h_r) \to_\delta (q',a,N)$ for all $q \in Q,h_l\in\Sigma_O'$

is an equivalent 2-PDA. Here, we assume that the Turing machine uses $\square \in \Sigma_O$ as blank symbol, both stacks start with a marker $\$\notin \Sigma_O$ (which is never removed) and $(q,a,h_l,h_r) \to_{\delta'} (q',l_1\dots l_i,r_1\dots r_j)$ means that $A_M$ consumes input $a$, switches states from $q$ to $q'$ and updates the stacks like so:

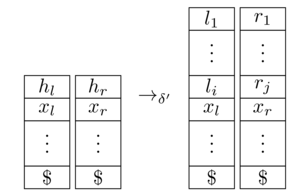
^[source]

It remains to show that $A_M$ enters a final state on $x \in \Sigma_I^*$ if and only if $M$ does so. This is quite clear by construction; formally, you have to translate accepting runs on $M$ into accepting runs on $A_M$ and vice versa.

Answer 2

At the beginning of Communicating and Mobile Systems: the Pi-Calculus by Robin Milner, there is a introduction on automata and how they can simulate each other so that they cannot be distinguished : Bisimulation. (cf Bisimulation on wikipedia)

I don't remember well, I should re-read the chapter, but there was a trouble with simulation and bisimulation that made them not sufficient for computational equivalences.

Thus Robin Milner introduces his Pi-Calculus and exposes it for the rest of the book.

Ultimately, in his last book The Space and Motion of Communicating Agents, you could have a look at Robin Milner's Bigraphs. They can model Automata, Petri nets, Pi-Calculus and other computational methodologies.

Answer 3

As far as I know, the only (or at least most common) way to do this is to compare the languages the machines/models accept. That's the whole point of Automata theory: it takes the vague concept of a problem or algorithm and turns it into a concrete mathematical set (i.e. a language) which we can reason about.

The easiest way to do this is, given an arbitrary machine/function from one model, to construct a machine from the second model that computes the same language. Odds are you'll use induction in the length of the expression, states in the machine, rules in the grammar, etc.

I haven't seen this done with Lambda and TMs (though I'm 99% sure it's possible), but I have definitely seen this kind of thing for proving equivalence of NFAs and Regular expressions. First you show a NFA which can accept any atom, then using induction, you make NFAs which accept the union/concatenation/Kleene-star of any smaller NFAs.

Then you do the opposite, to find an RE for any NFA.